Hi all. There is a problem.
I have tried to approximate a polynom of the third order
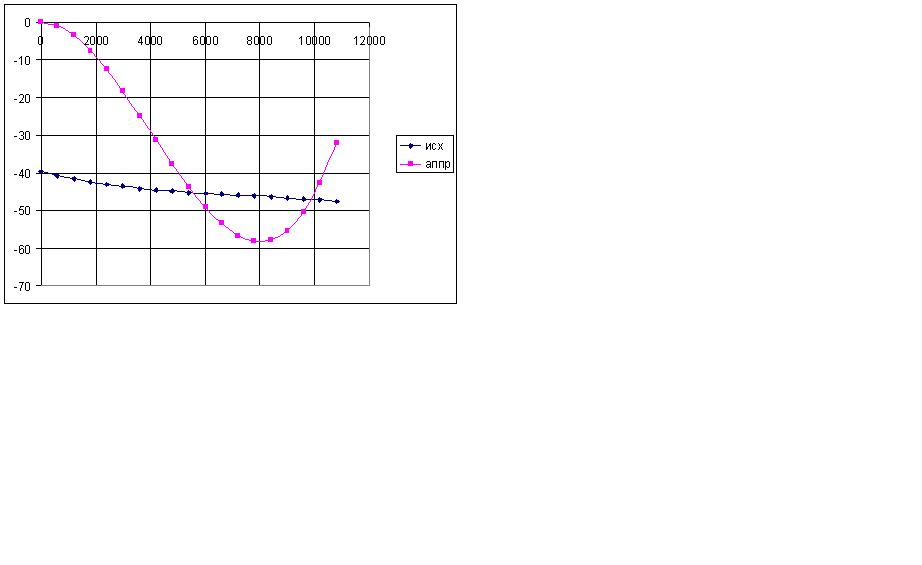
y:=-39.692 - 1.7902e-3*x + 1.8854e-7*x^2-8.4099e-12*x^3 by means of
a polynom of the third order. Used function LSFitLinear.
The result of approximation is resulted in drawing.
Attachment:
 m1.JPG [ 29.31 KiB | Viewed 7124 times ]
m1.JPG [ 29.31 KiB | Viewed 7124 times ]
Blue color - initial function. Lilac - result of approximation.
The example of approximation of a tangent resulted on a site works normally.
I use ALGLIB with Delphi 7.
In what the reason of such result?



